Case Interview Math (formulas, practice problems, tips)

(Article updated 17th October 2024)

Today we’re going to give you everything you need in order to breeze through math calculations during your case interviews. 

Becoming confident with math skills is THE first step that we recommend to candidates like Karthik, who got an offer from McKinsey. 

And one of the first things you’ll need to know are the 6 core math formulas that are used extensively in case interviews. 

Let’s dive in!

• Case interview math formulas
  • Must-know formulas
  • Optional formulas
  • Cheat sheet
• Practice questions
• Case math apps and tools
• Tips and tricks
• Practice with experts

Click here to practice 1-on-1 with MBB ex-interviewers

1. Case interview math formulas

1.1. Must-know math formulas

Here’s a summarised list of the most important math formulas that you should really master for your case interviews:

If you want to take a moment to learn more about these topics, you can read our in-depth article about finance concepts for case interviews.

1.2. Optional math formulas

In addition to the above, you may also want to learn the formulas below. 

Having an in-depth understanding of the business terms below and their corresponding formulas is NOT required to get offers at McKinsey, BCG, Bain and other firms. But having a rough idea of what they are can be handy.

EBITDA = Earnings Before Interest Tax Depreciation and Amortisation

EBIDTA is essentially profits with interest, taxes, depreciation and amortisation added back to it.

It's useful for comparing companies across industries as it takes out the accounting effects of debt and taxes which vary widely between, say, Meta (little to no debt) and ExxonMobil (tons of debt to finance infrastructure projects). More here.

NPV = Net Present Value

NPV tells you the current value of one or more future cashflows. 

For example, if you have the option to receive one of the two following options, then you could use NPV to choose the more profitable option:

• Option 1: receive $100 in 1 year and $100 in 2 years
• Option 2: receive $175 in 1 year

If we assume that the interest rate is 5% then option 1 turns out to be slightly better. You can learn more about the formula and how it works here.

Return on equity = Profits / Shareholder equity

Return on equity (ROE) is a measure of financial performance similar to ROI. ROI is usually used for standalone projects while ROE is used for companies. More here.

Return on assets = Profits / Total assets

Return on assets (ROA) is an alternative measure to ROE and a good indicator of how profitable a company is compared to its total assets. More here.

1.3 Case interview math cheat sheet

If you’d like to get a free PDF cheat sheet that summarises the most important formulas and tips from this case interview math guide, just click on the link below.

Download free pdf case interview math cheat sheet

2. Case interview math practice questions

If you’d like some examples of case interview math questions, then this is the section for you!

Doing math calculations is typically just one step in a broader case, and so the most realistic practice is to solve problems within the context of a full case.

So, below we’ve compiled a set of math questions that come directly from case interview examples published by McKinsey and Bain. 

We recommend that you try solving each problem yourself before looking at the solution. 

Now here’s the first question!

2.1 Payback period - McKinsey case example

This is a paraphrased version of question 3 on McKinsey’s Beautify practice case:

Note: take a moment to try solving this problem yourself, then you can get the answer under question 3 on McKinsey’s website. 

2.2 Cost reduction - McKinsey case example

This is a paraphrased version of question 2 on McKinsey’s Diconsa practice case:

Note: take a moment to try solving this problem yourself, then you can get the answer under question 2 on McKinsey’s website. 

2.3 Product launch - McKinsey case math example

This is a paraphrased version of question 2 on McKinsey’s Electro-Light practice case:

Note: take a moment to try solving this problem yourself, then you can get the answer under question 2 on McKinsey’s website. 

2.4 Pricing strategy - McKinsey case math example

This is a paraphrased version of question 3 on McKinsey’s Talbot Trucks practice case:

Note: take a moment to try solving this problem yourself, then you can get the answer under question 3 on McKinsey’s website. 

2.5 Inclusive hiring - McKinsey case math example

This is a paraphrased version of question 3 on McKinsey’s  Shops Corporation practice case:

Note: take a moment to try solving this problem yourself, then you can get the answer under question 3 on McKinsey’s website. 

2.6 Breakeven point - Bain case math example

This is a paraphrased version of the calculation portion of Bain’s Coffee Shop Co. practice case: 

Note: take a moment to try solving this problem yourself, then you can get the answer on Bain’s website.

2.7 Driving revenue - Bain case math example

This is a paraphrased version of the calculation part of Bain’s FashionCo practice case: 

Note: take a moment to try solving this problem yourself, then you can get the answer on Bain’s website.

3. Case math apps and tools

In the case math problems in the previous section, there were essentially 2 broad steps: 

1. Set up the equation
2. Perform the calculations

After learning the formulas earlier in this guide, you should be able to manage the first step. But performing the mental math calculations will probably take some more practice. 

Mental math is a muscle. But for most of us, it’s a muscle you haven’t exercised since high school. As a result, your case interview preparation should include some math training.

If you don't remember how to calculate basic additions, substractions, divisions and multiplications without a calculator, that's what you should focus on first.

In addition, Khan Academy has also put together some helpful resources. Here are the ones we recommend if you need an in-depth arithmetic refresher:

• Additions and subtractions
• Multiplications and divisions
• Percentages
• Scientific notation

Once you're feeling comfortable with the basics you'll need to regularly exercise your mental math muscle in order to become as fast and accurate as possible.

You might want to use some of the following resources. We haven't tested all of them, but some of the candidates we work with have used them in the past and found them helpful.

1. Preplounge's math tool. This web tool is very helpful to practice additions, subtractions, multiplications, divisions and percentages. You can both sharpen your precise and estimation math with it.
2. Victor Cheng's math tool. This tool is similar to the Preplounge one, but the user experience is less smooth in our opinion.
3. Mental math cards challenge app (iOS). This mobile app lets you work on your mental math easily on your phone. Don't let the old school graphics deter you from using it. The app itself is actually very good.
4. Mental math games (Android). If you're an Android user this one is a good substitute to the mental math cards challenge one on iOS.

4. Case interview math tips and tricks

4.1. Calculators are often not allowed in case interviews

If you weren’t aware of this rule already, then you’ll need to know this: 

Calculators are not usually allowed in case interviews. This applies to both in-person and virtual case interviews. And that’s why it’s crucial for candidates to practice doing mental math quickly and accurately before attending a case interview. 

And unfortunately, doing calculations without a calculator can be really slow if you use standard long divisions and multiplications. 

But there are some tricks and techniques that you can use to simplify calculations and make them easier and faster to solve in your head. That’s what we’re going to cover in the rest of this section. 

Let’s begin with rounding numbers.

4.2. Round numbers for speed and accuracy

The next 5 subsections all cover tips that will help you do mental calculations faster. Here’s an overview of each of these tips: 

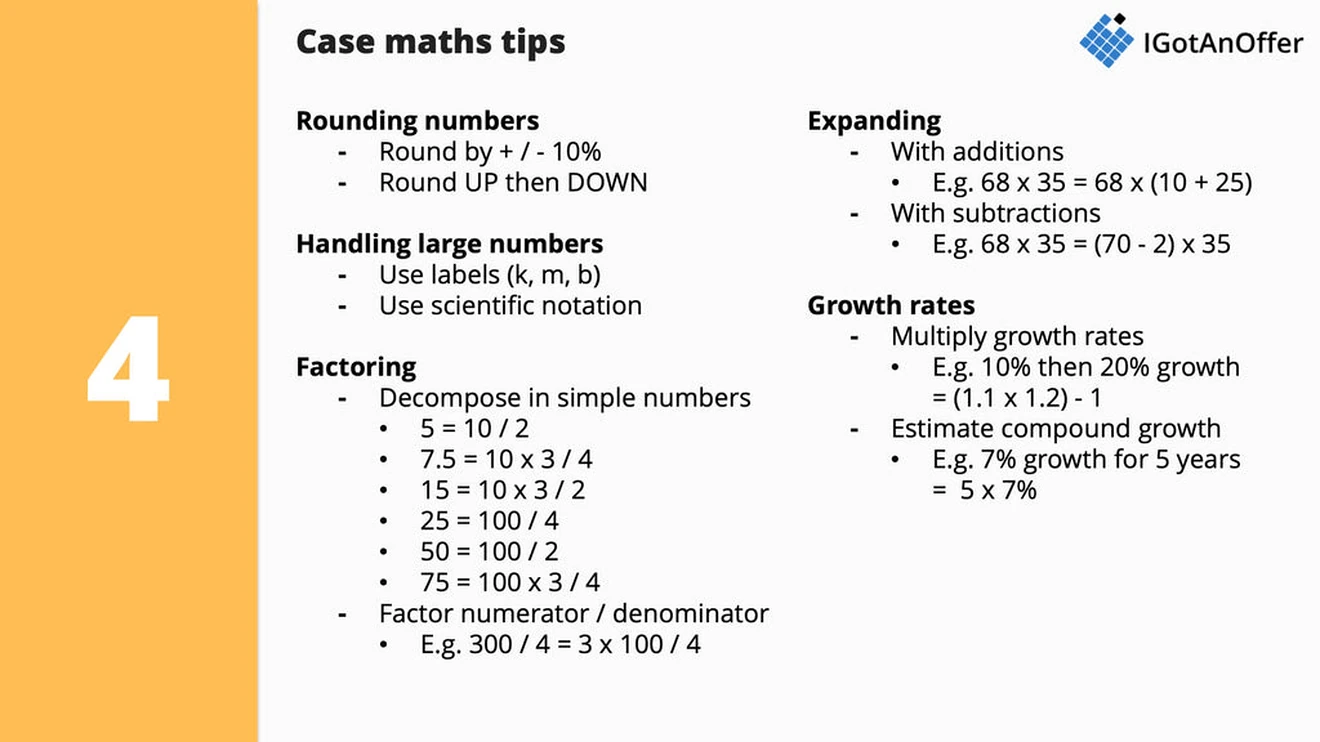
And the first one that we’ll cover here is rounding numbers. 

The tricky thing about rounding numbers is that if you round them too much you risk:

1. Distorting the final result
2. Or your interviewer telling you to round the numbers less

Rounding numbers is more of an art than a science, but in our experience, the following two tips tend to work well:

• We usually recommend that you avoid rounding numbers by more than +/- 10%. This is a rough rule of thumb but gives good results based on conversations with past candidates.
• You also need to alternate between rounding up and rounding down so the effects cancel out. For instance, if you're calculating A x B, we would recommend rounding A UP, and rounding B DOWN so the rounding balances out.

Note that you won't always be able to round numbers. In addition, even after you round numbers the calculations could still be difficult. So let's go through a few other tips that can help in these situations.

4.3. Abbreviate large numbers

Large numbers are difficult to deal with because of all the 0s. To be faster you need to use notations that enable you to get rid of these annoying 0s. We recommend you use labels and the scientific notation if you aren't already doing so.

Labels (k, m, b)

Use labels for thousand (k), million (m), and billion (b). You'll write numbers faster and it will force you to simplify calculations. Let's use 20,000 x 6,000,000 as an example.

• No labels: 20,000 x 6,000,000 = ... ???
• Labels: 20k x 6m = 120k x m = 120b

This approach also works for divisions. Let's try 480,000,000,000 divided by 240,000,000.

• No labels: 480,000,000,000 / 240,000,000 = ... ???
• Labels: 480b / 240m = 480k / 240 = 2k

Scientific notation

When you can't use labels, the scientific notation is a good alternative. If you're not sure what this is, you're really missing out. But fortunately, Khan Academy has put together a good primer on that topic here.

• Multiplication example: 600 x 500 = 6 x 5 x 102 X 102 = 30 x 104 = 300,000 = 300k
• Division example: (720,000 / 1,200) / 30 = (72 / (12 x 3)) x (104 / (102 x 10)) = (72 / 36) x (10) = 20

When you're comfortable with labels and the scientific notation you can even start mixing them:

• Mixed notation example: 200k x 600k = 2 x 6 x 104 x m = 2 x 6 x 10 x b = 120b

4.4. Use factoring to make calculations simpler

To be fast at math, you need to avoid writing down long divisions and multiplications because they take a LOT of time. In our experience, doing multiple easy calculations is faster and leads to less errors than doing one big long calculation.

A great way to achieve this is to factor and expand expressions to create simpler calculations. If you're not sure what the basics of factoring and expanding are, you can use Khan Academy again here and here. Let's start with factoring.

Simple numbers: 5, 15, 25, 50, 75, etc.

In case interviews some numbers come up very frequently, and it's useful to know shortcuts to handle them. Here are some of these numbers: 5, 15, 25, 50, 75, etc. 

These numbers are common, but not particularly easy to handle.

For instance, consider 36 x 25. It's not obvious what the result is. And a lot of people would need to write down the multiplication on paper to find the answer. However there's a MUCH faster way based on the fact that 25 = 100 / 4. Here's the fast way to get to the answer:

• 36 x 25 = (36 / 4) x 100 = 9 x 100 = 900

Here's another example: 68 x 25. Again, the answer is not immediately obvious. Unless you use the shortcut we just talked about; divide by 4 first and then multiply by 100:

• 68 x 25 = (68 / 4) x 100 = 17 x 100 = 1,700

Factoring works both for multiplications and divisions. When dividing by 25, you just need to divide by 100 first, and then multiply by 4. In many situations this will save you wasting time on a long division. Here are a couple of examples:

• 2,600 / 25 = (2,600 / 100) x 4 = 26 x 4 = 104
• 1,625 / 25 = (1,625 / 100) x 4 = 16.25 x 4 = 65

The great thing about this factoring approach is that you can actually use it for other numbers than 25. Here is a list to get you started:

• 2.5 = 10 / 4
• 5 = 10 / 2
• 7.5 = 10 x 3 / 4
• 15 = 10 x 3 / 2
• 25 = 100 / 4
• 50 = 100 / 2
• 75 = 100 x 3 / 4
• Etc.

Once you're comfortable using this approach you can also mix it with the scientific notation on numbers such as 0.75, 0.5, 0.25, etc.

Factoring the numerator / denominator

For divisions, if there are no simple numbers (e.g. 5, 25, 50, etc.), the next best thing you can do is to try to factor the numerator and / or denominator to simplify the calculations. Here are a few examples:

• Factoring the numerator: 300 / 4 = 3 x 100 / 4 = 3 x 25 = 75
• Factoring the denominator: 432 / 12 = (432 / 4) / 3 = 108 / 3 = 36
• Looking for common factors: 90 / 42 = 6 x 15 / 6 x 7 = 15 / 7

4.5. Expand numbers to make calculations easier

Another easy way to avoid writing down long divisions and multiplications is to expand calculations into simple expressions.

Expanding with additions

Expanding with additions is intuitive to most people. The idea is to break down one of the terms into two simpler numbers (e.g. 5; 10; 25; etc.) so the calculations become easier. Here are a couple of examples:

• Multiplication: 68 x 35 = 68 x (10 + 25) = 680 + 68 x 100 / 4 = 680 + 1,700 = 2,380
• Division: 705 / 15 = (600 + 105) / 15 = (15 x 40) / 15 + 105 / 15 = 40 + 7 = 47

Notice that when expanding 35 we've carefully chosen to expand to 25 so that we could use the helpful tip we learned in the factoring section. You should keep that in mind when expanding expressions.

Expanding with subtractions

Expanding with subtractions is less intuitive to most people. But it's actually extremely effective, especially if one of the terms you are dealing with ends with a high digit like 7, 8 or 9. Here are a couple of examples:

• Multiplication: 68 x 35 = (70 - 2) x 35 = 70 x 35 - 70 = 70 x 100 / 4 + 700 - 70 = 1,750 + 630 = 2,380
• Division: 570 / 30 = (600 - 30) / 30 = 20 - 1= 19

4.6. Simplify growth rate calculations

You will also often have to deal with growth rates in case interviews. These can lead to extremely time-consuming calculations, so it's important that you learn how to deal with them efficiently.

Multiply growth rates together

Let's imagine your client's revenue is $100m. You estimate it will grow by 20% next year and 10% the year after that. In that situation, the revenues in two years will be equal to:

• Revenue in two years = $100m x (1 + 20%) x (1 + 10%) = $100m x 1.2 x 1.1 = $100m x (1.2 + 0.12) = $100m x 1.32 = $132m

Growing at 20% for one year followed by 10% for another year therefore corresponds to growing by 32% overall.

To find the compound growth you simply need to multiply them together and subtract one: (1.1 x 1.2) - 1= 1.32 - 1 = 0.32 = 32%. This is the quickest way to calculate compound growth rates precisely.

Note that this approach also works perfectly with negative growth rates. Let's imagine for instance that sales grow by 20% next year, and then decrease by 20% the following year. Here's the corresponding compound growth rate:

• Compound growth rate = (1.2 x 0.8) - 1 = 0.96 - 1 = -0.04 = -4%

See how growing by 20% and then shrinking by 20% is not equal to flat growth (0%). This is an important result to keep in mind.

Estimate compound growth rates

Multiplying growth rates is a really efficient approach when calculating compound growth over a short period of time (e.g. 2 or 3 years).

But let's imagine you want to calculate the effect of 7% growth over five years. The precise calculation you would need to do is:

• Precise growth rate: 1.07 x 1.07 x 1.07 x 1.07 x 1.07 - 1 = ... ???

Doing this calculation would take a lot of time. Fortunately, there's a useful estimation method you can use. You can approximate the compound growth using the following formula:

• Estimate growth rate = Growth rate x Number of years

In our example:

• Estimate growth rate: 7% x 5 years = 35%

In reality if you do the precise calculation (1.075 - 1) you will find that the actual growth rate is 40%. The estimation method therefore gives a result that's actually quite close. In case interviews your interviewer will always be happy with you taking that shortcut as doing the precise calculation takes too much time.

4.7. Memorise key statistics

In addition to the tricks and shortcuts we’ve just covered, it can also help to memorise some common statistics. 

For example, it would be good to know the population of the city and country where your target office is located. 

In general, this type of data is useful to know, but it's particularly important when you face market sizing questions. 

So, to help you learn (or refresh on) some important numbers, here is a short summary:

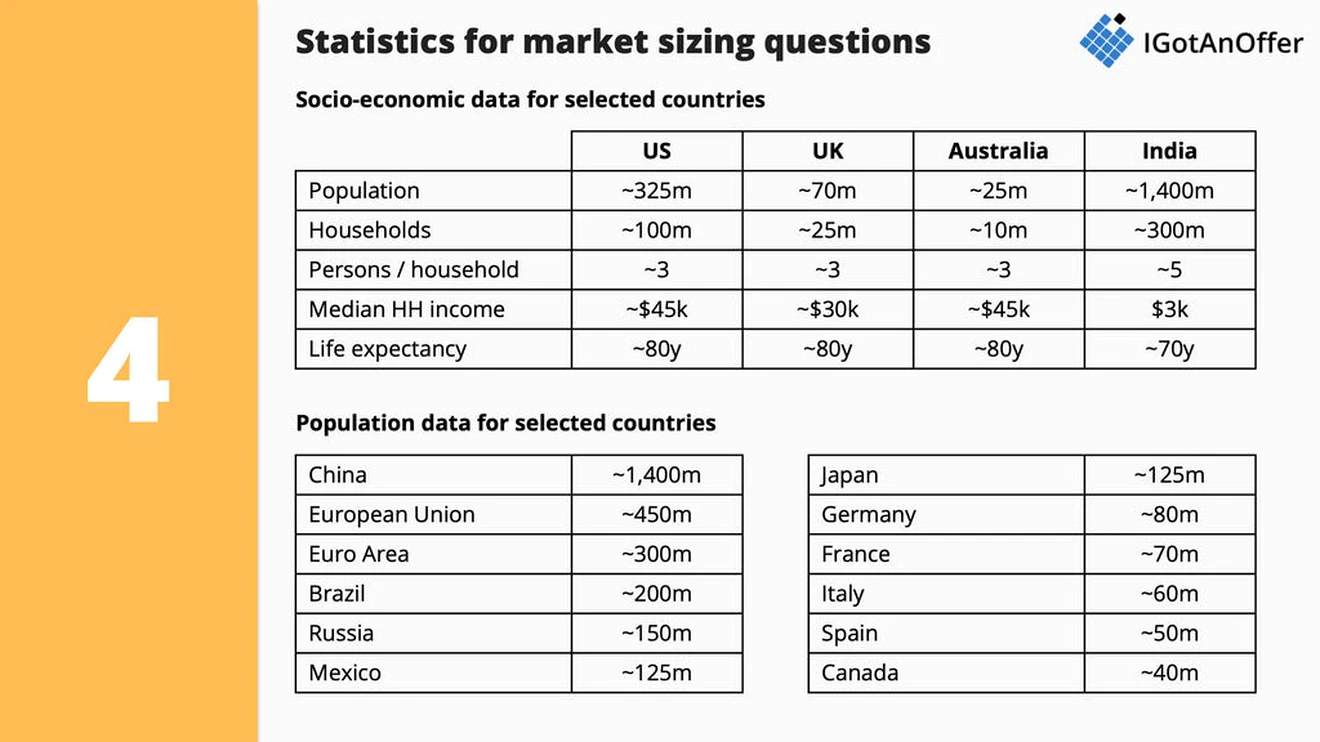
Of course this is not a comprehensive set of numbers, so you may need to tailor it to your own location or situation.  

5. Practising case interview maths

Sitting down and working through the math formulas we've gone through in this article is a key part of your case interview preparation. But it isn’t enough.

At some point, you’ll want to practice making calculations under interview conditions.

5.1 Practise with peers

If you have friends or peers who can do mock interviews with you, that's an option worth trying. It’s free, but be warned, you may come up against the following problems:

• It’s hard to know if the feedback you get is accurate
• They’re unlikely to have insider knowledge of interviews at your target company
• On peer platforms, people often waste your time by not showing up

For those reasons, many candidates skip peer mock interviews and go straight to mock interviews with an expert.

5.2 Practise with experienced MBB interviewers

In our experience, practising real interviews with experts who can give you company-specific feedback makes a huge difference.

Find a consulting interview coach so you can:

• Test yourself under real interview conditions
• Get accurate feedback from a real expert
• Build your confidence
• Get company-specific insights
• Learn how to tell the right stories, better.
• Save time by focusing your preparation

Landing a job at a top consulting company often results in a $50,000 per year or more increase in total compensation. In our experience, three or four coaching sessions worth ~$500 make a significant difference in your ability to land the job. That’s an ROI of 100x!

Click here to book case interview coaching with experienced MBB interviewers.